Thanks for sharing, Mo! I do not think humans need to have special status for one to prioritise interventions targeting humans. I estimate GiveWell's top charities may well be more cost-effective than interventions targeting animals due to effects on soil nematodes, mites, and springtails.

Thanks, Nick.

I guess AIM, ACE, AWF, and RP publicly explaining why they are not considering effects on soil animals would improve their reputation inside and outside the effective altruism community.

I guess the methodology RP used to obtain their mainline welfare ranges would imply higher welfare ranges for soil nematodes, mites, and springtails than the ones I estimated, so I think I am already adjusting downwards. My best guess is that I should adjust downwards even more, but that the expected change in the welfare of soil microorganisms, nematodes, mites, and springtails is still the major driver of the expected change in welfare caused by the vast majority of interventions.

Thanks for sharing your thoughts, Nick! I very much agree with your 1st point (not with the other points), but I guess effects on microorganisms, and soil nematodes, mites, and springtails are still the driver of the overall effect of the vast majority of the interventions. In any case, AIM, ACE, AWF, and RP use RP's mainline welfare ranges without adjusting downwards those of less complex species, and I believe this clearly implies that effects on microorganisms, and soil nematodes, mites, and springtails are the driver of the overall effect of the vast majority of interventions they assess. So I think AIM, ACE, AWF, and RP should still explain why they are not accounting for those effects.

Thanks for the post, Matthew!

(Also, if you go full Vasco and think the main impact of one’s meat consumption comes from its impact on insects, animals that roam about consume more food and decrease insect populations more).

I think the following replacements are generally beneficial due to increasing agricultural land:

• Plant-based foods, farmed shrimp, eggs, or farmed fish with poultry or pig meat.
• Poultry or pig meat with cheese.
• Cheese with beef.

Thanks for the comment, Ariel! That makes sense to me.

Thanks for the great points, Michael!

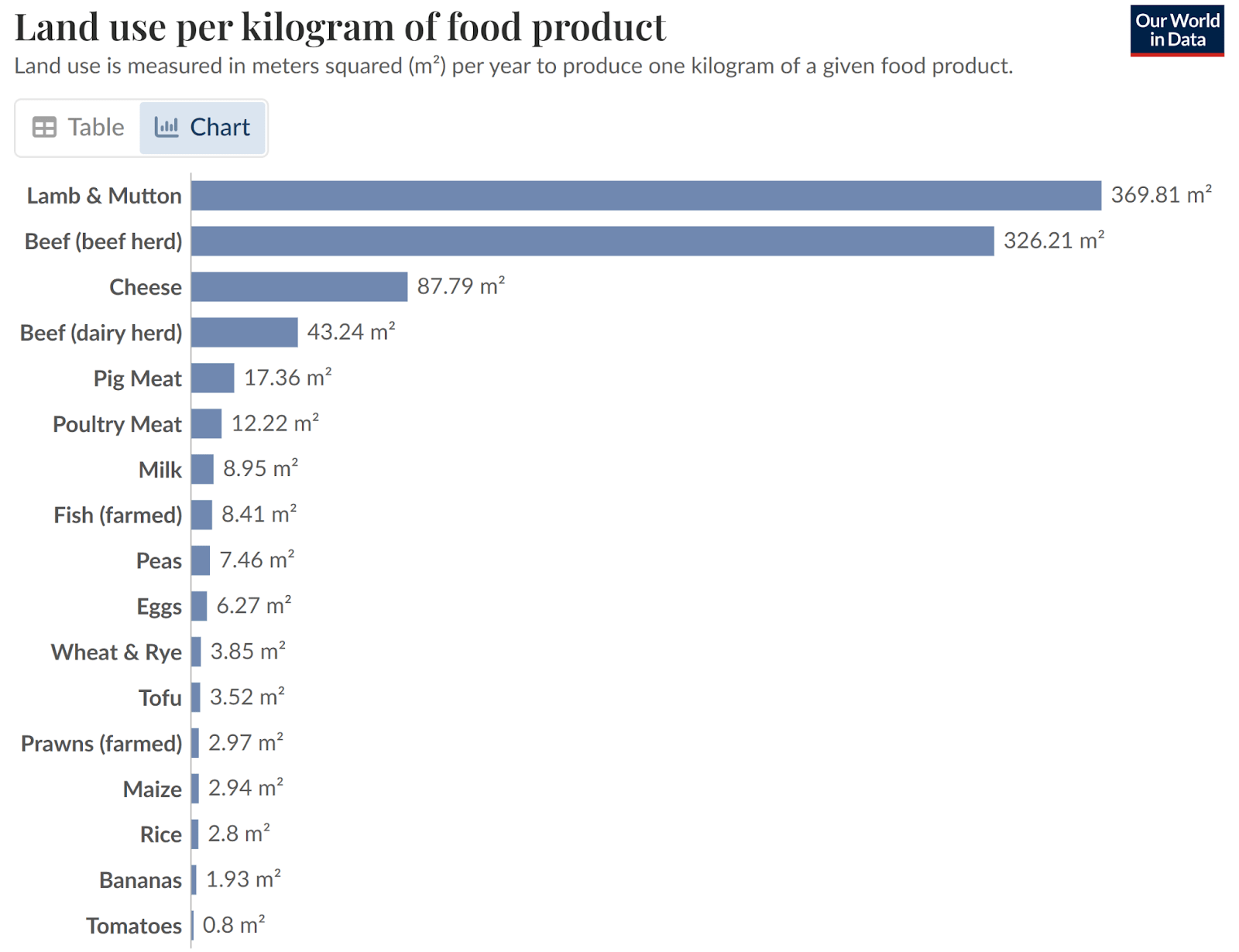
@Ailanthus, I am tagging you because I forgot to mention I recommend GiveWell's funds over the High Impact Philanthropy Fund (HIPF) from the Centre for Exploratory Altruism Research (CEARCH). I estimate the interventions funded by HIPF decrease agricultural land due to decreasing calorie consumption, despite decreasing human mortality, and therefore increasing human-years.

Yes, I estimated over 99.9 % of the benefits of donating to GiveWell's top charities come from reducing the number of soil animals.

I would say buying animal-based foods is in agreement with human values in the sense of increasing the welfare of both consumers and animals.

I would be surprised if there are cost-effective ways of advocating for decreasing agricultural land which decrease human welfare. The cost-effectiveness of advocating for an intervention is the cost-effectiveness of the intervention times the money moved to it as a fraction of the spending on advocating it, and this fundraising multiplier will tend to be much lower for advocy for supporting interventions which decrease human welfare.

I doubt that total human welfare is negative. I estimated only 6.37 % of people have negative lives. However, even if total human welfare was negative, I do not think it would make sense for superintelligent AI to kill all humans:

• I guess making human welfare positive would be not only more beneficial, but also cheaper, thus increasing human welfare more cost-effectively.
• Even if killing all humans was the most cost-effective way of increasing human welfare, I believe the overall effect of this would be driven by effects on soil nematodes, mites, and springtails, and that these would be harmed as a result of the decrease in agricultural-land-years caused by the extinction of humans.

I think increasing human-years via decreasing human mortality is generally more cost-effective than through increasing human fertility. GiveWell's top charities save a life for around 5 k$, 10 % of the lowest cost per additional birth of 50 k$ I found with a quick search.

I think effects on humans are smaller than those on soil animals, so I would focus on these whenever there are conflicts, but I am sceptical about finding cost-effective ways of helping soil animals that significantly harm humans.

I think desertification is beneficial because deserts, and xeric shrublands is the biome with the least soil nematodes, mites, and springtails by far, and my best guess is that these have negative lives, such that decreasing their population is good (although I am highly uncertain).

The cost-effectiveness of advocating for an intervention is the cost-effectiveness of the intervention times the money moved to the intervention as a fraction of the spending advocating for it. I think this fundraising multiplier would be very low for desertification efforts even if they decrease the living time of soil animals more cost-effectively than GiveWell's top charities, such that advocating for supporting these is more cost-effective.

RP's probability of sentience of crayfish (similar to lobsters) is only 1.54 (= 0.453/0.294) times RP's probability of sentient of black soldier flies (BSFs).

Thanks, Ailanthus. I would say my recommendation of supporting GiveWell's funds is very much in agreement with human values. In which sense do you think there is a conflict?