Hide table of contents

Comment Permalink

Thanks, Oscar. I do not know how much creatinine one should have in the blood to maximise the cognitive benefits. From the meta-analysis of Xu et al. (2024), it looks like the optimal supplementation is an open question (emphasis mine):

Despite these limitations, the results of this study provide promising evidence for creatine as a cognitive enhancer, particularly in improving memory and information processing speed. Notably, these findings specifically support creatine monohydrate as an effective form of supplementation. This evidence offers a scientific basis for the application of creatine in cognitive enhancement and provides direction for future research. Future studies should aim to optimize creatine supplementation strategies, including exploring the optimal dosage, supplementation duration, and long-term effects, to maximize its cognitive benefits. Additionally, further research is needed to elucidate the mechanisms by which creatine affects cognitive function and to investigate its interactions with other cognitive interventions, such as cognitive training and other nutritional supplements.

It is still very early days. It is not even clear whether creatine supplementation improves overall cognitive function. Below is the forest plot of the 6 studies meta-analysed in Xu et al. (2024).

Below is a scatter plot with the effect size and daily dosage of the above studies. A positive value favours creatine. I think the legend of the horizontal axis above should have [Con] on the left, and [Cre] (creatine) on the right.

There is basically no correlation. The coefficient of determination is 0.961 % (and the slope is negligibly negative, -0.00906 d/g).

In terms of how much creatine one can take, from the systemic review of Kreider et al. (2017):

[...] There is no compelling scientific evidence that the short- or long-term use of creatine monohydrate (up to 30 g/day for 5 years) has any detrimental effects on otherwise healthy individuals or among clinical populations who may benefit from creatine supplementation.

So I assume it is fine for one to experiment with daily dosages higher than the conventional 5 g/d.

One can determine the optimal daily dosage speculating about the function describing the benefits. For my assumption that the benefits are proportional to the logarithm of 1 plus the daily dosage in g/d, the benefits in $/year are 10.6*10^3/ln(1 + 3)*ln(1 + "daily dosage in g/d") = 7.65*10^3*ln(1 + "daily dosage in g/d"). The cost in $/year is "cost in $/year/(g/d)"*"daily dosagr in g/d". So the net benefits in $/year are 7.65*10^3*ln(1 + "daily dosage in g/d") - "cost in $/year/(g/d)"*"daily dosage in g/d". The derivative of this with respect to the daily dosage is 7.65*10^3/(1 + "daily dosage in g/d") - "cost in $/year/(g/d)", which is 0 for a daily dosage in g/d of 7.65*10^3/"cost in $/year/(g/d)" - 1. This is the dosage for which the net benefits are maximum because their 2nd derivative with respect to the daily dosage, -7.65*10^3/(1 + "daily dosage in g/d")^2, is always negative. For my cost of 31.7 $/year/(g/d) (= 95.2/3), the optimum daily dosage is 240 g/d (= 7.65*10^3/31.7 - 1). I think this is way too high because I am underestimating the diminishing returns of the benefits. My function implies they grow indefinitely with ln(1 + "daily dosage in g/d"), but they will eventually stagnate, decrease and become negative (harmful) for sufficiently large daily dosages, even if my assumption is a good approximation for small dosages.

See in context

Break-even analysis of creatine supplementation

by Vasco Grilo🔸

Dec 29 20244 min read 9

39

ForecastingCognitive enhancementCost-benefit analysisDietary changeFermi estimateProductivityMedicine

Frontpage

Break-even analysis of creatine supplementation

The views expressed here are my own.

Summary

I estimate the improvement in cognitive function caused by creatine supplementation is worth it for a net income above 15.5 k$/year.
I think creatine supplementation is beneficial, although I do not know if cost-effective, ignoring improvements in overall cognitive function. According to the systematic review of Kreider and Stout (2021), “it can be concluded that creatine supplementation has several health and therapeutic benefits throughout the lifespan”.

Break-even analysis

I estimate the improvement in cognitive function caused by creatine supplementation is worth it for a net income above 15.5 k$/year. I calculate this from the ratio between:

A cost of creatine supplementation of 119 $/year, respecting:
- Powder, which is 116 $/year cheaper than capsules under my assumptions.
- A financial cost of 0.214 $/d for 0.0713 $/g and 3 g/d.
- A time cost of 0.167 $/d for 30 s/d and 20 $/h.
A relative increase in net income caused by creatine supplementation of 3 g/d of 0.897 %. I get this multiplying:
- An improvement in the overall cognitive function caused by creatine supplementation of “1 IQ [intelligence quotient] point”, as calculated in Sandkühler et al. (2023) for improvements in Raven’s Advanced Progressive Matrices (RAPM) for a “daily supplementation of 5 g for 6 weeks”.
  - Xu et al. (2024) meta-analysed 6 randomised controlled trials assessing the effect of creatine supplementation on overall cognitive function, including Sandkühler et al. (2023), and the standardised mean difference respecting this is 32.4 % (= 0.11/0.34) of that linked to their meta-analytic estimate (see Figure 3).
  - Sandkühler et al. (2023), which was linkposted on the EA Forum, is a randomised, double-bind, placebo-controlled, and preregistered trial. None of the other 5 studies meta-analysed in Xu et al. (2024) with respect to overall cognitive function were preregistered.
  - I assume the improvement in cognitive function caused by creatine supplementation does not decay over time. From the systemic review of Kreider et al. (2017), "significant health benefits may be provided by ensuring habitual low dietary creatine ingestion (e.g., 3 g/day [my assumed dosage]) throughout the lifespan".
- A dosage adjustment factor of 77.4 %. I obtain this for my assumed lower dosage of 3 g/d guessing the improvement in the overall cognitive function is proportional to the logarithm of 1 plus the daily dosage in g/d.
- An increase in net income of 1.16 % per additional IQ point. This is the effect of national IQ on education- and age-adjusted wages of immigrants in the United States found by Jones and Schneider (2010) (see Table 1).
  - The result is in line with other literature. "We show that a country’s average IQ score is a useful predictor of the wages that immigrants from that country earn in the United States, whether or not one adjusts for immigrant education. Just as in numerous microeconomic studies, 1 IQ point predicts 1% higher wages, suggesting that IQ tests capture an important difference in crosscountry worker productivity".

Discussion

Xu et. al (2024) estimated a 95 % confidence interval for the improvement in the overall cognitive function caused by creatine supplementation of 0.0980 to 0.570 pooled standard deviations (Hedge’s g), or 29.3 % (= 0.0980/0.334) to 171 % (= 0.570/0.334) of their best estimate. Using these to adjust my mainline result, the improvement in cognitive function caused by creatine supplementation is worth it for a net income above 9.06 k (= 15.5*10^3/1.71) to 52.9 k$/year (= 15.5*10^3/0.293).

I wanted to estimate the benefits of creatine supplementation from studies looking into its effect on net income, but I did not find them. So I relied on the effect of creatine supplementation on cognitive function, and of increasing IQ on wages.

Sandkühler et al. (2023) “found no indication that our vegetarian participants benefited more from creatine than our omnivore participants (in fact, the creatine effect was smaller in vegetarians than omnivores to a non-statistically significant extent). This is in line with Solis et al. [22, 24] who did not find a difference in brain creatine content between omnivores and vegetarians”.

I think creatine supplementation is beneficial, although I do not know if cost-effective, ignoring improvements in overall cognitive function. According to the systematic review of Kreider and Stout (2021), “it can be concluded that creatine supplementation has several health and therapeutic benefits throughout the lifespan”.

I liked Antonio et al. (2021), “Common questions and misconceptions about creatine supplementation: what does the scientific evidence really show?”.

Based on our evidence-based scientific evaluation of the literature, we conclude that:
Creatine supplementation does not always lead to water retention.
Creatine is not an anabolic steroid.
Creatine supplementation, when ingested at recommended dosages, does not result in kidney damage and/or renal dysfunction in healthy individuals.
The majority of available evidence does not support a link between creatine supplementation and hair loss / baldness.
Creatine supplementation does not cause dehydration or muscle cramping.
Creatine supplementation appears to be generally safe and potentially beneficial for children and adolescents.
Creatine supplementation does not increase fat mass.
Smaller, daily dosages of creatine supplementation (3-5 g or 0.1 g/kg of body mass) are effective. Therefore, a creatine ‘loading’ phase is not required.
Creatine supplementation and resistance training produces the vast majority of musculoskeletal and performance benefits in older adults. Creatine supplementation alone can provide some muscle and performance benefits for older adults.
Creatine supplementation can be beneficial for a variety of athletic and sporting activities.
Creatine supplementation provides a variety of benefits for females across their lifespan.
Other forms of creatine are not superior to creatine monohydrate.

39 Reactions

More posts like this

Comments9

Sorted by

New & upvoted

Click to highlight new comments since: Today at 5:54 AM

MichaelDickensDec 30 20246

A time cost of 0.0417 $/d for 7.5 s/d and 20 $/h.

Nitpick: I just timed myself taking creatine and it took me 42 seconds.

(My process consists of: take creatine and glass out of cabinet; scoop creatine into glass; pour tap water into glass; drink glass; put creatine and glass back into cabinet.)

Agreed that creatine passes a cost-benefit analysis.

Vasco Grilo🔸Dec 30 20245

Thanks for the comment, Michael. I did not time myself, but 7.5 s/d is my guess for the difference between taking 5 capsules (including one of creatine; capsules are 23.5 $/year cheaper under my assumptions) and the 4 I already take after lunch (D3, Omega 3, iodine and a multivitamin; I take B12 before breakfast). I have all the supplements in the same place, so it would not take me much time to take another capsule.

Vasco Grilo🔸Jan 262

capsules are 23.5 $/year cheaper under my assumptions

My financial cost of capsules was 3 times as high as it should be. I thought the 3 g of creatine mentioned in the nutritional composition were the amount per capsule, whereas they were the amount per 3 capsules. Now powder looks 116 $/year cheaper than capsules for a time cost of 30 s/d (instead of my original assumption of 20 s/d).

I have updated to reflect using powder with the updated time cost. The updated break-even net income is 15.5 k$/year, 1.46 (= 15.5*10^3/(10.6*10^3)) times my original value.

[anonymous]Dec 30 20244

Isn't it even more important for people on vegan diets (e.g. me)? According to Claude:

- - - - - -

Creatine supplementation is particularly relevant for vegans for several biological reasons:

The primary reason is that creatine is naturally found almost exclusively in animal products, particularly meat and fish. The human body can synthesize creatine from amino acids, but vegans typically have lower baseline creatine levels in their muscles since they don't consume any dietary sources.

Your body's natural creatine synthesis requires three amino acids: glycine, arginine, and methionine. While vegans can get these amino acids from plant sources, the total creatine levels tend to be lower than in omnivores. Research has shown that vegetarians and vegans typically have about 50-60% of the creatine muscle stores of people who eat meat.

Creatine is important because it helps:
- Produce ATP (energy) for short, intense activities
- Improve muscle strength and power output
- Support cognitive function
- Aid in muscle recovery and growth

For vegans specifically, supplementing with creatine can help bridge this nutritional gap and bring their creatine levels up to optimal ranges. Studies have shown that vegans and vegetarians often experience more pronounced benefits from creatine supplementation compared to meat-eaters, likely because they're starting from a lower baseline.

The standard recommended dose (5g daily) works well for vegans, and creatine monohydrate is vegan-friendly as it's typically synthesized from non-animal sources.

Vasco Grilo🔸Dec 30 20242

Thanks, Yanni. My hypothesis was also that vegetarians and vegans would benefit more from creatine supplementation, but it looks like there is no clear evidence for that. Below is the abstract of the systematic review of Kaviani et al. (2020) (emphasis mine).

Background: Creatine monohydrate is a nutritional supplement often consumed by athletes in anaerobic sports. Creatine is naturally found in most meat products; therefore, vegetarians have reduced creatine stores and may benefit from supplementation. Objective: to determine the effects of creatine supplementation on vegetarians. Data sources: PubMed and SPORTDiscus. Eligibility criteria: Randomized controlled trials (parallel group, cross-over studies) or prospective studies. Participants: Vegetarians. Intervention: Creatine supplementation. Study appraisal and synthesis: A total of 64 records were identified, and eleven full-text articles (covering nine studies) were included in this systematic review. Results: Creatine supplementation in vegetarians increased total creatine, creatine, and phosphocreatine concentrations in vastus lateralis and gastrocnemius muscle, plasma, and red blood cells, often to levels greater than omnivores. Creatine supplementation had no effect on brain levels of phosphocreatine. Creatine supplementation increased lean tissue mass, type II fiber area, insulin-like growth factor-1, muscular strength, muscular endurance, Wingate mean power output, and brain function (memory and intelligence) in vegetarian participants. Studies were mixed on whether creatine supplementation improved exercise performance in vegetarians to a greater extent compared to omnivores. Limitations: Studies that were reviewed had moderate–high risk of bias. Conclusions: Overall, it appears vegetarian athletes are likely to benefit from creatine supplementation.

The 2nd sentence I highlighted above refers to "exercise performance", but it is also unclear whether vegetarians and vegans benefit more from creatine supplementation with respect to cognitive function. From my post:

Sandkühler et al. (2023) “found no indication that our vegetarian participants benefited more from creatine than our omnivore participants (in fact, the creatine effect was smaller in vegetarians than omnivores to a non-statistically significant extent). This is in line with Solis et al. [22, 24] who did not find a difference in brain creatine content between omnivores and vegetarians”.

[anonymous]Dec 30 20244

Thanks for clarifying! I’ve been taking 5mg for a couple of years. How much do you take? 5mg is a generic recommendation so I’m considering experimenting with higher doses.

Vasco Grilo🔸Dec 31 20242

You are welcome! I have actually never taken creatine, and did the analysis to figure out whether I should^[1]. I am planning to start with 3 g/d. Here are some thoughts on optimising creatine supplementation.

^{^}
Currently, I take B12, D3, Omega 3, iodine (following the advice of NutritionFacts.org), and a multivitamin (to cover any eventual gaps left by following a plant-based diet; for example, I do not think I consume enough iron excluding that I take via the multivitamin).

OscarD🔸Dec 31 20244

Thanks for writing this up, I just looked back at the results of a generic blood test measuring many different things I did earlier in the year and I had a creatinine value of 0.82 (the reference range was given as 0.7-1.3).
I haven't looked through the literature you cited, do you happen to know if I am already in the healthy range whether it is still helpful to be supplementing, or if it is bad to go over 1.3 if I do supplement?

Vasco Grilo🔸Dec 31 20244

Despite these limitations, the results of this study provide promising evidence for creatine as a cognitive enhancer, particularly in improving memory and information processing speed. Notably, these findings specifically support creatine monohydrate as an effective form of supplementation. This evidence offers a scientific basis for the application of creatine in cognitive enhancement and provides direction for future research. Future studies should aim to optimize creatine supplementation strategies, including exploring the optimal dosage, supplementation duration, and long-term effects, to maximize its cognitive benefits. Additionally, further research is needed to elucidate the mechanisms by which creatine affects cognitive function and to investigate its interactions with other cognitive interventions, such as cognitive training and other nutritional supplements.

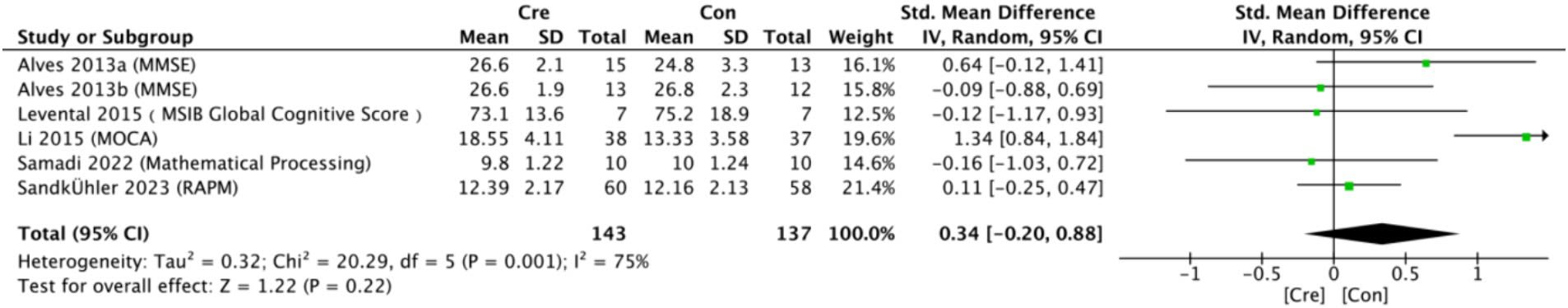
It is still very early days. It is not even clear whether creatine supplementation improves overall cognitive function. Below is the forest plot of the 6 studies meta-analysed in Xu et al. (2024).

There is basically no correlation. The coefficient of determination is 0.961 % (and the slope is negligibly negative, -0.00906 d/g).

In terms of how much creatine one can take, from the systemic review of Kreider et al. (2017):

[...] There is no compelling scientific evidence that the short- or long-term use of creatine monohydrate (up to 30 g/day for 5 years) has any detrimental effects on otherwise healthy individuals or among clinical populations who may benefit from creatine supplementation.

So I assume it is fine for one to experiment with daily dosages higher than the conventional 5 g/d.